The ‘variable’ constant

One of the most fascinating predictions of quantum electrodynamics (QED), is the existence of a state of minimum energy, the vacuum state, which is full of particles, namely the particle-antiparticle pairs that exist for a very short time and are therefore called virtual. These pairs, which are formed in vacuum in the same way air bubbles in a pot of water on the stove, are mainly composed of light particles like electron and anti-electron (positron). Occasionally, however, other types of heavier particle pairs can be formed, like muon-antimuon or quark-antiquark (quarks are the elementary constituents of the proton and neutron), or possibly pairs of still unknown particles. As the bubbles forming at the bottom of the pot and rising to the water surface, these virtual particles produce tangible effects to the physical processes involving real elementary particles.

Concerning electrical properties, the vacuum behaves in the same way as a common insulating material. In the presence of an electrical charge, the spatial distribution of particles and anti-particles warps due to the electric field of the charge. Particles with opposite charge will be attracted, while those with the same charge will be pushed away.

This vacuum polarization effect has an important implication. If we design an experiment to measure the electric charge of a particle, the result of the experiment is a function of how close our probe is to the charge: the effective charge decreases at a great distance, while it increases as we get closer to the position of the original charge. In technical terms, this is called ‘running’ of the electromagnetic field coupling constant α, also known as the fine structure constant. This behavior is responsible for the fact that the value of α corresponding to the mass of the vector boson Z₀ (about 90 GeV) is 1/128, instead of about 1/137, which is usually the value when the energy involved is very small.

But inside the quantum vacuum there are all kinds of particles, so we should wonder about its properties in the context of other interactions of Nature. We found out that things can be different from the electromagnetic interaction. For example, with regard to the color interaction, experienced by quarks and responsible for the nuclear force, this kind of screening effect works in the opposite way: an elementary charge of color, for example, a quark, attracts virtual charges of the same color, repelling those of the opposite color. Such behavior of the quantum vacuum means that, contrary to the electromagnetic constant, the strong coupling constant grows as the distance increases and vanishes when quarks are in contact with each other. This phenomenon, known as asymptotic freedom, was predicted in 1972 by David Gross, David Politzer and Frank Wilczek, who were rewarded with the Nobel Prize in 2004.

Going back to the evolution of the electromagnetic coupling constant, there is another important aspect to take into account. In fact, while the screening effect due to leptons (electrons, muons and tauons) is known with high precision, the same cannot be said of the contribution of the quark-antiquark pairs. In particular, this contribution cannot be calculated in the range of energies of the order of GeV, therefore, indirect determinations must be used.

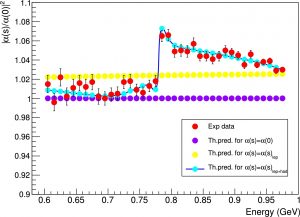
The KLOE-2 collaboration, analyzing the data of the electron-positron conversion process into muon pairs, measured for the first time the running of α in the energy region below 1 GeV, highlighting in a spectacular way (with a statistical significance larger than five standard deviations) the contribution of virtual quark-antiquark pairs to the vacuum polarization.

Pictured in the graph the running of the square electromagnetic coupling constant, normalized at its value at zero energy (α (0) ≃ 1/137), as a function of the muon pair’s invariant mass. Red dots represent experimental data; turquoise and yellow dots represent, respectively, the expected behavior when considering quarks and leptons, and when considering leptons only. Purple dots do not receive any contribution from the vacuum polarization.

The article, in press in the journal Physics Letters B, is freely available at: https://arxiv.org/pdf/1609.06631.pdf (Graziano Venanzoni)